Introduction
A straight angle is a fundamental concept in geometry, forming the basis for many other geometrical principles. It is crucial for understanding various mathematical problems and solutions. This blog post aims to provide a comprehensive understanding of straight angles, including their definition, properties, applications, and more.
Definition of a Straight Angle
A straight angle is an angle that measures exactly 180 degrees. It is formed when the two rays or line segments constituting the angle extend in exactly opposite directions, creating a straight line. The keyword “straight angle” is used to emphasize the perfect straightness of the angle formed.
Properties of a Straight Angle
The properties of a straight angle are unique and distinct. One of the primary properties is that the straight angle is always 180 degrees, making it a linear pair with no curvature. Additionally, the arms of a straight angle are collinear, which means they lie on the same straight line. Understanding these properties helps in identifying and working with straight angles in various geometric problems.
Visual Representation of a Straight Angle
Visualizing a straight angle can aid in better understanding. When two rays originate from a common point and extend in opposite directions, forming a line, they create a straight angle. This visual representation is crucial for recognizing straight angles in geometric figures and diagrams.
Mathematical Significance of Straight Angles
In mathematics, straight angles play a significant role in the study of lines, angles, and shapes. They are essential in the formation of linear equations and in understanding the properties of parallel and perpendicular lines. The concept of a straight angle is foundational for various mathematical theorems and proofs.
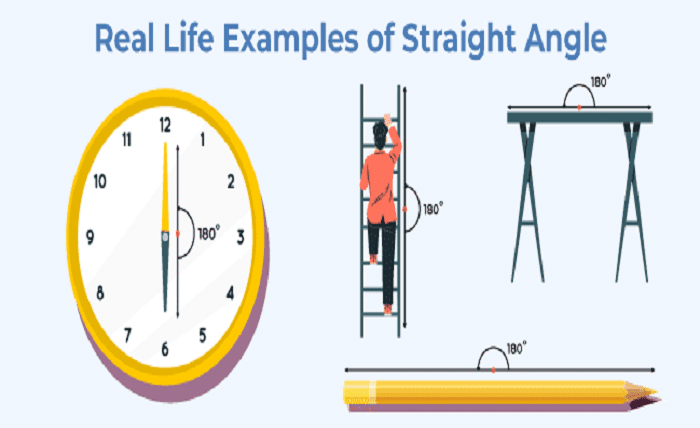
Real-World Examples of Straight Angles
Straight angles are not just theoretical; they are prevalent in the real world. Examples include the hands of a clock at 6:00, the angle formed by a flat surface, and the alignment of roads and pathways. Recognizing straight angles in everyday life helps in appreciating their practical applications.
Straight Angles in Geometry
In geometry, straight angles are fundamental in constructing and understanding different shapes and figures. They are used in the formation of polygons, determining parallelism and perpendicularity, and in the study of congruent and similar shapes. Straight angles also aid in solving various geometric problems and proofs.
Straight Angles and Linear Pairs
A linear pair is formed when two adjacent angles add up to a straight angle, totaling 180 degrees. Understanding the relationship between linear pairs and straight angles is crucial for solving problems involving adjacent angles and their measures. This relationship is a key concept in geometry.
Measuring a Straight Angle
Measuring a straight angle is straightforward, as it always equals 180 degrees. Using a protractor or geometric tools, one can easily measure and confirm the angle’s straightness. Accurate measurement is essential for precise geometric constructions and problem-solving.
Applications of Straight Angles in Engineering and Architecture
Straight angles are extensively used in engineering and architecture. They are fundamental in designing structures, ensuring stability and symmetry. Engineers and architects use the concept of straight angles to create plans, layouts, and frameworks for buildings, bridges, and other constructions.
Teaching Straight Angles in Education
Educators emphasize the importance of straight angles in the curriculum. Teaching straight angles involves explaining their definition, properties, and applications. Interactive activities and visual aids are used to help students grasp the concept and apply it in various mathematical problems.
Conclusion
In conclusion, a straight angle is a crucial concept in geometry, with significant mathematical and real-world applications. Understanding its definition, properties, and various uses helps in solving complex geometric problems and appreciating its presence in everyday life. Whether in education, engineering, or daily observations, the straight angle remains a fundamental aspect of our understanding of geometry.
FAQs
1. What is a straight angle?
A straight angle is an angle that measures exactly 180 degrees, formed by two rays extending in opposite directions, creating a straight line.
2. How is a straight angle different from other angles?
A straight angle is unique because it always measures 180 degrees, unlike other angles which can vary in measurement. It forms a straight line with no curvature.
3. Can a straight angle be less than 180 degrees?
No, a straight angle is defined as exactly 180 degrees. Any angle less than 180 degrees is considered an acute or obtuse angle, not a straight angle.
4. Where can we see straight angles in real life?
Straight angles can be observed in various real-life situations, such as the hands of a clock at 6:00, the edges of a flat surface, and the alignment of roads and pathways.
5. Why is understanding straight angles important in geometry?
Understanding straight angles is important because they are fundamental to various geometric principles, including the study of lines, shapes, and angles. They are essential for solving geometric problems and constructing accurate diagrams.





